Optipedia • SPIE Press books opened for your reference.
Immersion Lithography
Excerpt from Field Guide to Optical Lithography
Resolution
If the air between the lens and the wafer is replaced with water, the optical invariant says that the angles of light inside the resist will be the same. There are two impacts on lithography: the maximum possible angle of light that can reach the resist is increased (increasing the maximum potential resolution), and the phase of that light is changed, causing an improvement in depth of focus.
The maximum value of the optical invariant will be limited by the material in the film stack above and including the resist with the smallest refractive index. If one of the layers is air (with a refractive index of 1.0), this will become the material with the smallest refractive index and the maximum possible value of the invariant will be 1.0. If the air is replaced with a fluid of a higher refractive index, but still smaller than the index of the photoresist, the maximum possible value of the invariant will be nfluid, and the maximum possible angle of light inside the resist will be greater: sinθmax,resist =
nfluid/nresist. At a wavelength of 193 nm, resists have refractive indices of about 1.7 and water has a refractive index of about 1.44. The fluid does not make the angles of light larger, but it enables those angles to be larger. If one were to design a lens to emit larger angles, immersion lithography will allow those angles to propagate into the resist. The numerical aperture of the lens (defined as the maximum value of the invariant n sinθ that can pass through the lens) can be made to be much larger using immersion lithography, with the resulting improvements in resolution one would expect.
Immersion lithography is now in use and is expected to allow lenses to be made with numerical apertures greater than 1.0. Lenses with NAs above 1.2 or 1.3 seem likely. If an immersion fluid with a refractive index closer to that of the photoresist can be found, numerical apertures of up to 1.5 might be possible.
Depth of Focus
For a given diffraction order (and thus a given angle of the light inside the resist), the angle of the light inside an immersion fluid will be less than if air were used. These smaller angles will result in smaller optical path differences between the various diffracted orders when out of focus, and thus a smaller degradation of the image for a given amount of defocus. In other words, for a given feature being printed and a given numerical aperture, immersion lithography will provide a greater depth of focus (DOF). The high NA version of the Rayleigh depth of focus criterion (which assumes we are imaging a small binary pattern of lines and spaces of pitch p) is
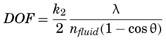
where nfluid sinθ = λ/p. Combining these equations, one can see how immersion will improve the depth of focus of a given small feature:

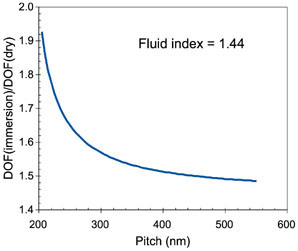
C. A. Mack, Field Guide to Optical Lithography, SPIE Press, Bellingham, WA (2006).
View SPIE terms of use.

