Optipedia • SPIE Press books opened for your reference.

Explanation of triplets from Fundamental Optical Design

The simplest design that is capable of correcting all of the seven Seidel aberrations over a wide field of view is the Cooke triplet. H. Dennis Taylor invented this in 1893, using the advances of Seidel's theory. It is named after the optical company in York, England, for which Taylor worked at the time, Cooke and Sons (later to become Cooke, Troughton and Sims). The lens is described in two very interesting United States patents, Nos. 540,132 (1895) and 568,053 (1896). Taylor's designs, despite their antiquity, are close to optimum for the aperture and field he intended, given the glass types available in his day. The triplet uses two of the principles of a good design. First, the Petzval sum is corrected by the use of spaced positive and negative lenses, as described in Chapter 9 on telephoto lenses. Secondly, it has approximate front-back symmetry about a central stop, to control the odd-order aberrations, coma, distortion, and transverse color.

Seidel theory

From the designer's point of view, the triplet (Fig. 10.1) is interesting because the initial analysis can be carried out using thin-lens theory, as will be briefly outlined below.

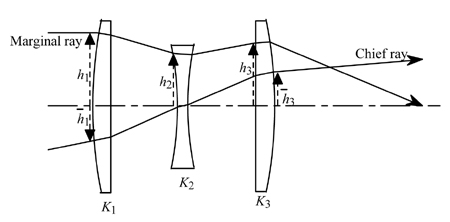
For the sake of this discussion we will assume that the lens thicknesses are fixed. In practice, the lens thicknesses of a triplet are not useful as design parameters. Of course, the lenses must be thick enough to be mechanically rigid and easy to mount. On the other hand, they must not be too thick if glass material costs or weight are factors governing the design. Typically, lens thicknesses are chosen to be between 5% and 10% of the glass diameter, depending on the type of lens, the manufacturer, and its power.

As in all designs, choice of glass types is important, but we assume at this stage that a preliminary choice of three glasses has been made. The effect of changing glass types will be shown in Sec. 10.3.

Having made these assumptions, there are now eight variables available. Two of them are the spaces, d₁ and d₂, between the three lenses (the back focal length to the image is treated as a quantity to be calculated, rather than as a parameter). There are also the curvatures of the six surfaces, which, for this analysis, are best considered as three powers, K₁, K₂, K₃, and three shape factors. These are the eight variables that we must employ to control the seven Seidel aberrations and the focal length.

Another factor is the position of the stop. In the classical Cooke triplet photographic objective, the stop is just behind the central negative lens. For the thin-lens analysis, we can assume the stop to coincide with the second lens, although in practice, since the iris diaphragm of a camera must be located at this point, there will need to be mechanical clearance between the aperture stop and the glass component. For a well-corrected design, however, a small movement of the stop will not change the aberrations by more than a small amount.

For a given choice of three glass types, the equations for the power, the Petzval sum, and the longitudinal and transverse color (the three shape-independent aberrations) become

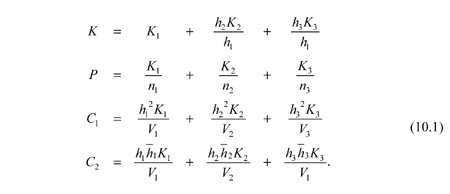
Note that if the stop is at the second lens, there is further simplification, since the second term in the formula for C₁ vanishes. Note also that in solving these four equations, it is not desirable to make P exactly zero, since exact correction of field curvature results in higher lens powers and increased higher-order aberrations. A typical value for P is about 0.35 K.

The four equations for the power and the three shape-independent aberrations can be rewritten in terms of the five parameters, K₁, K₂, K₃, d₁, and d₂. The extra variable permits a range of solutions that can be used in the way outlined below.

This leaves the four shape-dependent aberrations (S₁, S₂, S₃, and S₅) and only three shape factors to control them. Of course, if we bend the outer lenses, which are not in contact with the stop, all four aberrations will change. The simplest procedure is to correct S₃ and S₅ by choice of lens shapes of the outer components, and then to bend the second lens, which will only change
S₁ and S₂. In general, we can always find a shape to correct S₂; however, the shape that is best for correcting S₂ will not also correct S₁. However, by returning to the first four equations and using the spare variable there to create a range of different solutions, one can be found that gives an acceptable value of S₁ when the combination of shapes gives zero S₂. In this way, we can design the triplet to correct all of the Seidel aberrations.

As an example, contributions of each of the lenses in a thin-lens design are listed in Table 10.1. We can see that for each of the even aberrations, S₁, S₃, S₄, and C₁, the outer, positive lenses have a positive coefficient, and the inner, negative, lens has a negative coefficient.

Of the odd aberrations, distortion and lateral color are balanced by contributions of opposite sign from the first and the third lens; the aperture stop is so close to the second lens, the chief ray height there is small making it only a small contribution to the total S₅ and the total C₂. Mainly, a balance between the contributions of the first and second lenses corrects coma. It should be noted that the signs in this table of the Seidel coefficients of the odd aberrations S₂, S₅, and C₂ are arbitrary, since they depend on the sign of the Lagrange invariant, H.

The analytical design of a triplet is possible, but it is difficult. Triplets are probably the most complex systems that can be designed from the first principles described in this procedure. One of the attractions of this analytical approach is that it leads to the discovery of multiple solutions, in some cases. There are, for some pairs of glass types, two solutions that correct all the Seidel aberrations. The analytic approach will find all of the solutions. These days, in practice, if a triplet design were wanted, we would start from an existing design, and optimize!