Optipedia • SPIE Press books opened for your reference.

Soliton Fiber Lasers

While picosecond pulse generation in bulk lasers can dominantly rely on a saturable absorber action, dispersive and nonlinear effects cannot be ignored in fiber lasers even in the multipicosecond domain. Conceptually, the simplest approach leads to the soliton fiber laser, where the circulating pulses are quasi-soliton pulses.

For passive mode locking, artificial saturable absorbers based on the Kerr nonlinearity are often utilized. One possibility is to exploit nonlinear polarization rotation. Here, the polarization state in some length of fiber undergoes a complicated evolution, which depends on the optical power due to self- and cross-phase modulation. Last, the pulses are sent through a polarizing element, which converts the polarization changes to changes of power transmission.

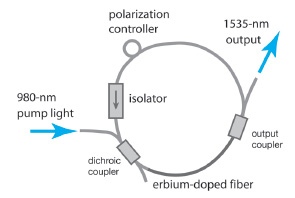
In the laser resonator, as shown above, the optical isolator serves as the polarizing element. The polarization controller is adjusted such that the peak of a pulse experiences overall lower losses than the pulse wings. Unfortunately, the optimum adjustment is not only influenced by any bending of the fiber, but also depends on the temperature. Therefore, such lasers are not environmentally stable: they need a carefully temperature-stabilized environment, or occasional tweaking.

Another kind of artificial saturable absorber is the nonlinear fiber loop mirror, which can be integrated into a figure-eight fiber laser (Ref. Duling 1991) as shown below.

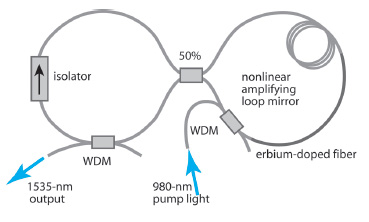
The actual laser resonator is seen on the left side, whereas the ring on the right side is a nonlinear amplifying fiber loop. Pulses from the laser resonator are split into two copies, propagating through the nonlinear loop in opposite directions. These pulses experience different nonlinear phase shifts, as one is first amplified in the erbium-doped fiber and then propagates through a longer piece of nonlinear fiber, while the other one propagates through that fiber with a lower energy. Therefore, the interference condition for recombining the two pulses at the fiber coupler in the middle becomes power-dependent: for a certain power level, much of the power can be sent downward in the laser resonator.

A figure-eight laser can be made with polarization-maintaining fiber so that it becomes environmentally stable. However, this implies significant manufacturing efforts because the polarization axes at many fiber joints need to be aligned.

Another possibility is to use a SESAM, possibly in fiber-coupled form, for mode locking.

Limitations of Soliton Fiber Lasers

The pulse duration of a soliton fiber laser is typically a few picoseconds, and the soliton pulse energy is in the picojoule domain. For shorter pulse durations, with correspondingly higher soliton pulse energy, the soliton periods quickly become too short, and the pulse formation becomes unstable due to too-high nonlinear phase shifts per round-trip. When this regime is approached, the pulse spectrum exhibits significant Kelly sidebands, as shown below.

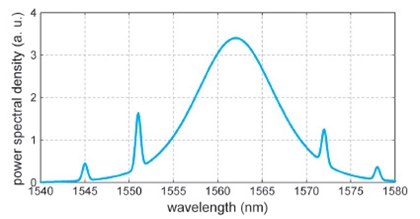
For pulse durations well below 1 ps, such effects become very difficult to handle, even with a strong saturable absorber.

In principle, large mode area fibers can be used for reducing nonlinear effects. However, this allows only for higher pulse energies, not for shorter pulses, as the dependence of the soliton period on the pulse duration is not fundamentally changed. Also, the use of such specialty fibers restricts the choice of fiber-optic components, making it more difficult to develop an all-fiber setup.

For shorter pulse durations and higher pulse energies, it is often necessary to use more complicated mode-locking techniques.