Optipedia • SPIE Press books opened for your reference.
Integrating Sphere
Excerpt from Field Guide to Illumination
Integrating spheres produce illumination that has extremely uniform radiance and irradiance. An integrating sphere is a hollow spherical shell coated on the inside with a highly reflecting diffuse coating. The projected solid angle from any point on a sphere to any element of area on the sphere is the same, regardless of location. This fact combined with the diffuse coating and the multiple reflections cause any light introduced into the sphere to produce uniform irradiance on and radiance of the wall of the sphere. A hole or "port" in the sphere allows this uniform illumination to be used in an optical system.
| The radiance at the exit of an integrating sphere extends to a full hemisphere (π projected steradians). The irradiance at the wall of an integrating sphere is incident from a full hemisphere. |
The radiance, L, of the wall of an integrating sphere generated by flux, Φ, introduced into the sphere is
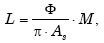
where As is the area of the complete sphere wall, and M is the "sphere multiplier," which is equal to the average numbers of reflections in the sphere. The multiplier, M, is
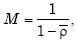
where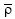 is the average reflectance of the wall of the sphere, counting the holes as areas of zero reflectance.
is the average reflectance of the wall of the sphere, counting the holes as areas of zero reflectance.
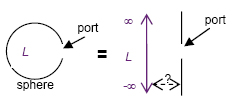 A good working model of an integrating sphere is to consider the port to be a hole in a wall, and, at a totally arbitrary distance behind it, another wall of infinite extent and radiance, L.
A good working model of an integrating sphere is to consider the port to be a hole in a wall, and, at a totally arbitrary distance behind it, another wall of infinite extent and radiance, L.
A. V. Arecchi, T. Messadi, and R. J. Koshel, Field Guide to Illumination, SPIE Press, Bellingham, WA (2007).
View SPIE terms of use.

