Optipedia • SPIE Press books opened for your reference.
Airy Disk explanation from Field Guide to Geometrical Optics
Excerpt from Field Guide to Geometrical Optics
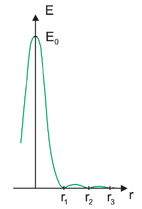
Because of diffraction from the system stop, an aberration-free optical system does not image a point to a point. An Airy disk is produced having a bright central core surrounded by diffraction rings.
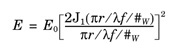
where r is the radial coordinate, J1 is a Bessel function, and f /#W is the imagespace working f /#.
| Radius r | Peak E | Energy in Ring (%) | |
| Central maximum | 0 | 1.0 E0 | 83.9 |
| First zero r1 | 1.22λf⁄#W | 0.0 | |
| First ring | 1.64λf⁄#W | 0.017 E0 | 7.1 |
| Second zero r2 | 2.24λf⁄#W | 0.0 | |
| Second ring | 2.66λf⁄#W | 0.0041 E0 | 2.8 |
| Third zero r3 | 3.24λf⁄#W | 0.0 | |
| Third ring | 3.70λf⁄#W | 0.0016 E0 | 1.5 |
| Fourth zero r4 | 4.24λf⁄#W | 0.0 |
The diameter of the Airy disk (diameter to the first zero) is
D = 2.44λf ⁄#W
| In visible light λ ≈ 0.5 μm and D≈f⁄#W in μm |
The Rayleigh resolution criterion states that two point objects can be resolved if the peak of one falls on the first zero of the other:
Resolution = 1.22λf ⁄#W
The angular resolution is found by dividing by the focal length (or image distance):
Angular resolution=α=1.22λ ⁄ DEP
Citation:
View SPIE terms of use.
J. E. Greivenkamp, Field Guide to Geometrical Optics, SPIE Press, Bellingham, WA (2004).
View SPIE terms of use.
Excerpt from

